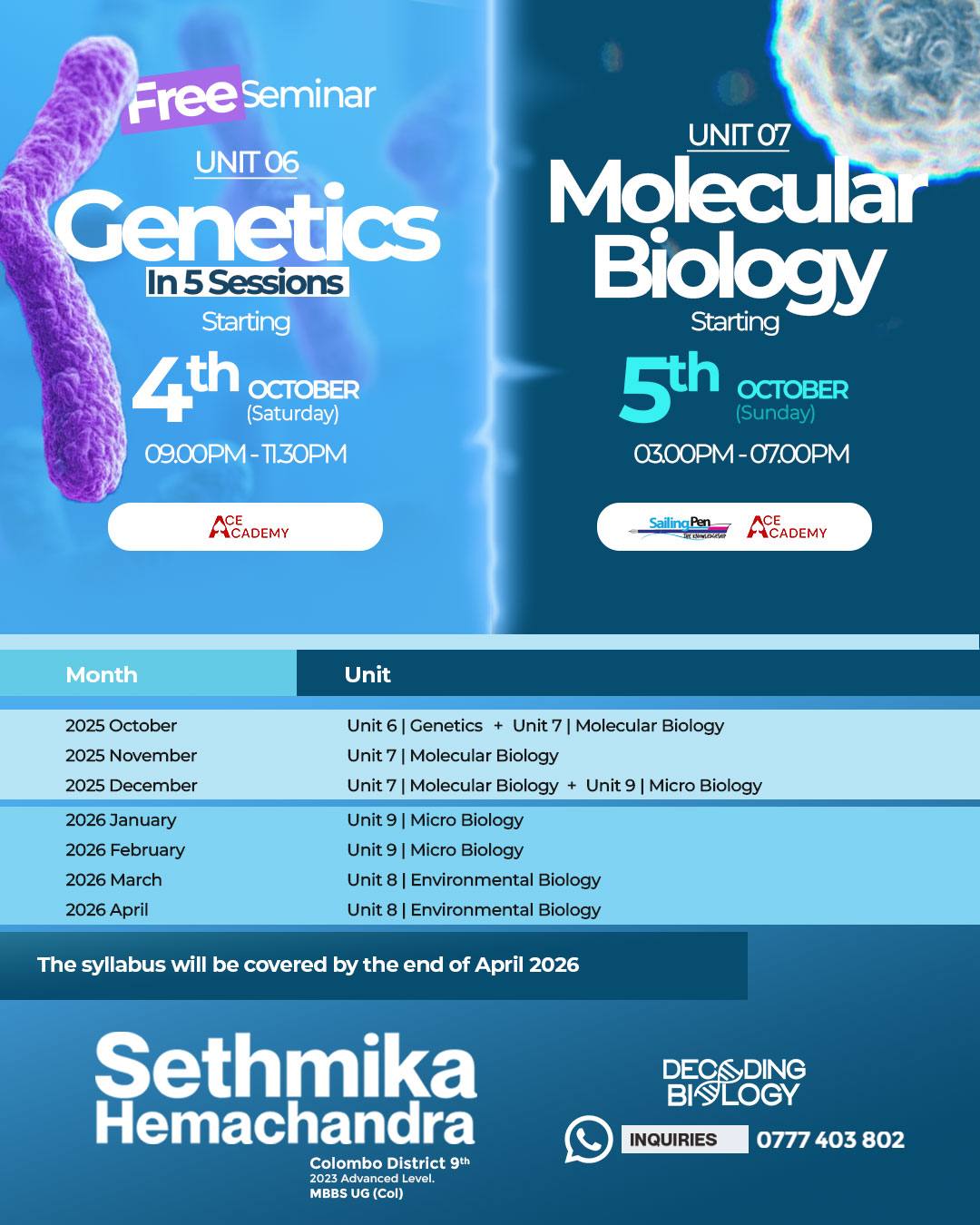
Coplanar Forces | ඒකතල බල - Comprehensive Study Pack - By Sudheera Peiris
Purchase Coplanar Forces Study Pack For Advanced Level 2024 Batch
- 14 Users Enrolled
- Created by: Sudheera Peiris
- Last Updated: 10th July 2024
- English/සිංහල
- 5000
7000
Having trouble purchasing or accesing the course? Contact Us or checkout out our Support Page
Overview
Don't know how to join ZOOM meetings or watch recordings? Contact Us or find out how by watching this video.
|
Spot Test 21 Assignment - English (PDF)
|
|||
|
Spot Test 21 Assignment - Sinhala (PDF)
|
|||
|
Coplanar Forces | ඒකතල බල - Tutorial English
|
|||
|
Coplanar Forces | ඒකතල බල - Tutorial Sinhala
|
|
Coplanar Forces | ඒකතල බල - Day 01
|
|||
|
Coplanar Forces | ඒකතල බල - Day 02
|
|||
|
Coplanar Forces | ඒකතල බල - Day 03
|
|||
|
Coplanar Forces | ඒකතල බල - Day 04
|
|||
|
Coplanar Forces | ඒකතල බල - Day 05
|
|||
|
Spot Test 21 Discussion - Coplanar Forces | ඒකතල බල - Day 06
|
Description
Coplanar Forces | ඒකතල බල Comprehensive Study Pack
- 05 Recordings (Theory / Paper Discussions / Past Paper Discussions)
- 1 Assingment (Spot Test 21)
- 1 Assingments Discussion (Spot Test 21)
- Tutorial (Both Mediums)
Advanced Level Combined Maths theory classes conducted by Mr. Sudheera Peiris.
Purchase Coplanar Forces Study Pack, For Advanced Level 2024 Batch
This bundle is a collection of Coplanar Forces Theory Classes conducted for 2024 Advanced Level Students. Please note that you are purchasing Recordings of live sessons conducted previously. By the end of this study pack you will learn the entire thoery coming under the Coplanar Forces unit.
Importance of Studying Coplanar Forces
-
Engineering Applications: Coplanar forces are forces that lie in the same plane. In engineering disciplines such as civil engineering, mechanical engineering, and structural engineering, structures often experience forces acting in a single plane. Understanding coplanar forces is crucial for designing stable and efficient structures, analyzing the behavior of mechanical components, and ensuring safety in engineering projects.
-
Equilibrium Analysis: Coplanar forces obey the principles of equilibrium, which means that the vector sum of all forces in the plane is zero if the system is in a state of static equilibrium. By studying coplanar forces, engineers and physicists can analyze structures and systems to determine whether they are in equilibrium or if they are subject to unbalanced forces that may cause movement or deformation.
-
Vector Analysis: Coplanar forces can be represented as vectors with magnitude and direction. Studying coplanar forces involves vector analysis techniques such as vector addition, decomposition, and resolution. These techniques are fundamental in understanding how multiple forces interact within a system and how they contribute to overall equilibrium or motion.
-
Free Body Diagrams (FBD): FBDs are graphical representations used to analyze forces acting on a body or a system. Studying coplanar forces often involves creating FBDs to visualize and analyze the forces acting on each component within the system. By drawing FBDs, engineers and physicists can systematically analyze the effects of external loads, internal forces, and reactions on structures and components.
-
Real-World Applications: Coplanar forces are prevalent in various real-world scenarios, such as bridges, buildings, machines, and vehicles. By studying coplanar forces, engineers can optimize designs, improve structural integrity, and ensure the safety and reliability of structures and mechanical systems. Coplanar force analysis is also essential in fields such as biomechanics, where understanding the forces acting on human joints and muscles helps in designing ergonomic equipment and analyzing human movement.
Q: What are the extra benefits of purchusing this bundle?
- Mr.Sudheera is a skilled teacher who has 8 years of teaching experience in Combined Maths and is well equipped to offer the best quality support
- Get your doubts clarified easily in the live class.
- You can post doubts on the WhatsApp group to be clarified by the teacher.
- Assistive technical team support to help you out.
Recently Added Courses
About the Instructor
Mr. Sudheera Peiris is an experienced and dedicated mathematics educator with a passion for teaching and inspiring students. With a background in mathematics and a solid understanding of pedagogical principles, he has been instrumental in helping students develop a strong foundation in math and excel in their academic pursuits.
He holds a Bachelor's degree in Mathematics from University of Colombo, where he graduated with honors.
With over Seven years of teaching experience, He has had the opportunity to teach a wide range of grade levels and student abilities. He has successfully implemented various teaching methodologies and differentiated instruction to accommodate diverse learning styles and help students grasp complex mathematical concepts.